2. Losses vs. flooding type and depth

Thames-Coromandel Civil Defence supplied colour-coded flood impact maps for the 'high impact' settlements. These showed that approximately 35 % of affected properties (about 300 within the 'high impact' settlements) suffered flooding of just their land, 37 % had flooded basements and/or outbuildings, and 28 % suffered house flooding above the floors of the living areas.

Documenting specific causes of damage (e.g., saturation of textiles and cladding, sedimentation, shear damage due to flow velocity and water column weight, impact from carried debris, flotation) is beyond the scope of this study. Collection of data on flooding type was considered during the design of the surveys. However the survey was conducted over a year after the event, so it was considered that recollection of details of the range of loss parameters (items damaged vs. how each was damaged) would likely be cloudy to the respondent. A more lengthy questionnaire would have detrimentally affected the return rate, resulting in a smaller sample if these details had been asked. No centralised data on the nature of flooding or damage was systematically captured at the time of the event, beyond impact maps (an example of one such map is given in Figure 5b).

The depth to which a house is flooded affects the number and types of items that are damaged. For example, flooding as little as 1 centimetre deep would damage flooring, but have little effect on anything that is not within a centimetre of the floor. Conversely flooding greater than 1 metre deep would be expected to damage all tables and bench tops and anything below or on top of these. Flooding above two metres deep would damage virtually everything in that room.

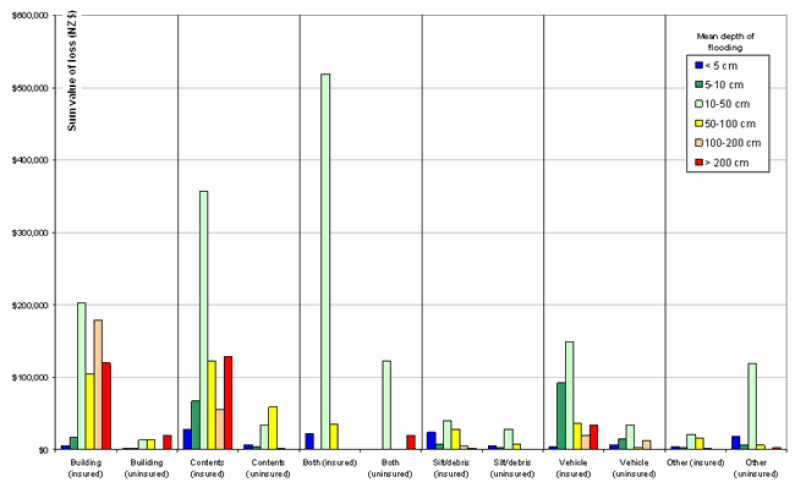
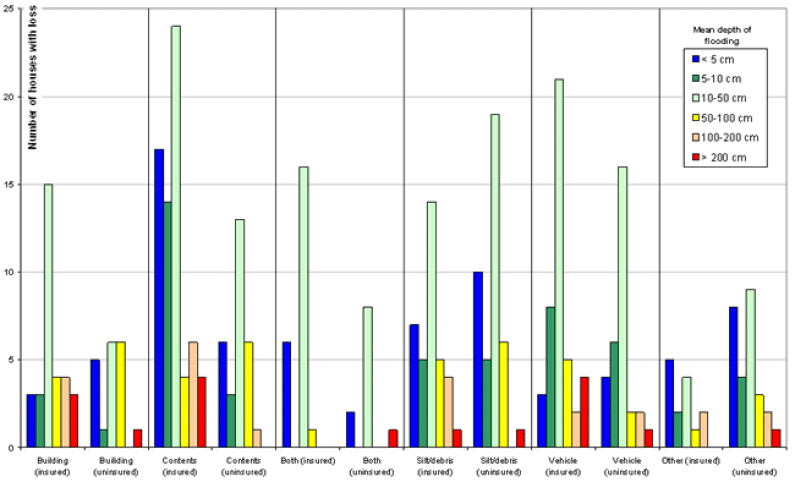
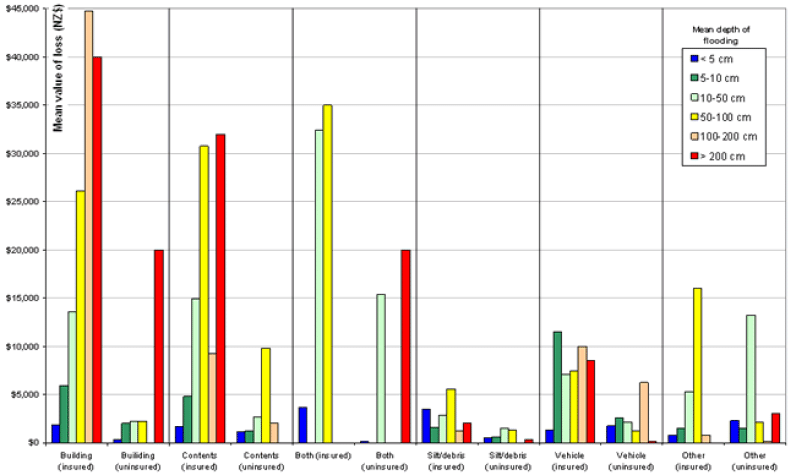
The following analysis and figures are based on data presented in Tables A3.4, A3.6 and A3.8. The mean depth of household flooding was computed for each property from estimated values given in survey responses for living room, bedroom, kitchen, bathroom, basement/cellar, hallway/landing and garage. These were then aggregated into depth brackets: < 5 cm, 5-10 cm, 10-50 cm, 50-100 cm, 100-200 cm and over 200 cm depth. Information, including the total aggregated loss, the number of cases with loss and the mean loss for each loss type (building/structure, contents, both building/structure and contents, removal of silt/debris, vehicles/boats/caravans/trailers, other) in each depth bracket were then computed and are plotted in Figures 6 through 8.

The total combined loss (Fig. 6) is greatest for houses with mean flooding depths of 10-50 cm for all insured loss types, and the majority of uninsured loss types Uninsured building and contents losses, when the respondent treated them separately from one another, were greatest with deeper flooding. This is partly a function of the number of houses flooded within each of the depth brackets (Fig. 7), which across the board is also highest for 1-50 cm depth. However there is also, in the cases of most loss types, a steady increase in mean loss with increasing mean flooding depth, up to 50 cm depth (Fig. 8).

The first point that is important to note from these figures is that, while the number of houses flooded to < 5 cm mean depth is proportionately higher than for the 5-10 cm bracket (for all but vehicle losses), the mean loss value for the < 5 cm bracket is the overall lowest for all costs other than silt and debris cleanup. This suggests that, as long as flooding is less than 5 cm mean depth, the mean value of losses will be disproportionately low. However, silt and debris cleanup costs are higher in more instances (possibly because with such shallow flooding flooring can be cleaned rather than needing to be replaced, thus avoiding a contents loss).

The second important point is that, for non-vehicle losses, the mean loss per house (Fig. 8) is particularly high for all depth brackets over 50 cm. Therefore, losses were a lot lower than if more properties had been flooded to > 50 cm mean depth (there were relatively few properties in this bracket, Fig. 7).

The trends for vehicle loss differ from those for household losses. Vehicle total losses (Fig. 6) are very much in proportion to the number of properties flooded to that average depth (Fig. 7). This is reflected in the relatively constant average vehicle loss (Fig. 8) for all depths > 5 cm. Vehicles on properties flooded to an average depth of < 5 cm had relatively very low average loss values (presumably because these flood waters were not deep enough to easily enter the vehicle's passenger compartment).

In summary:

• Household mean losses are disproportionately low when mean flooding in that house is 5 cm or less deep (cleanup of silt/debris may be possible in many cases, reducing permanent loss of contents).
• Average losses rise relatively proportionately to mean flooding depth for depths from 5 to 50 cm (through the ranges of height of beds, lounge furniture, TVs etc.).
• Household mean losses are disproportionately high when mean flooding in that house is more than 50 cm deep (reaching the heights for table and bench tops and anything stored on top of, or above these).
• Vehicle mean losses jump on properties with mean household flooding above 5 cm deep, and then remain relatively constant, even when the vehicle sits on a property with mean flooding over the height of the vehicle roof (the control may be the height of the base of the vehicle floor).

The largest combined overall losses in the Weather Bomb event were overwhelmingly in the 10-50 cm depth bracket, but this is partly because this was the most common mean depth of flooding for a flooded house.

If mean household flooding depths > 50 cm had been more common, the losses could have been an order of magnitude greater (other than for vehicle losses, which should have remained at similar levels). Conversely, if mean flooding had rarely exceeded 5 cm depth, losses would have been an order of magnitude less (including vehicle losses).

It would be useful to test a range of bracket cutoffs to determine more exact boundary values for key jumps in loss (e.g., vehicle mean loss may actually rise most dramatically at 4 or 6 cm, rather than the 5 cm used here). Such analysis would require an order of magnitude larger sample of loss cases than was collected here. The relatively low number of loss events (all n < 25) in each depth/loss-type class means that the margin of error (20 % at n=25) is too large for the dataset to be reliably used to determine differences of a few centimetres in the depth bracket boundaries.